
Copyright 1995 by Campbell R. Harvey. All rights reserved. No part of this lecture may be reproduced without the permission of the author.
Latest Revision: November 2, 1995.
Would you rather receive $100 today -- or next year? Obviously, you would want the money today. You could bank the money today and it would be worth more than $100 next year. Hence, time is an important consideration when valuing money.
We will explore the answer to two questions: What is the value today of $100 received next year
and what is the value next year of $100 received today? The first questions asks for a value today
and is known as
First, we need to establish some notation:

The effective periodic interest rate, i, equals the nominal interest rate, R, divided by the number of compounding periods annually:
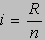
The effective (or true) annual interest rate, r is the annually compounded interest rate which equals the effective periodic rate, i, compounded n times per year.
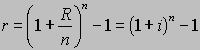
Note as n becomes large (many compounding periods per year), the effective annual interest rate becomes:
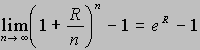
where e is the natural exponent, e is approximately 2.718. The proof
to this proposition is available.
We will prove that
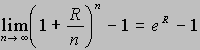
In the special case that R=0, the result is obvious. Consider the cases when R is not
equal to zero. Take the natural logarithm of the function on the right-hand side (RHS).
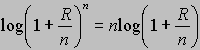
By multiplying and dividing by R and noting that the logarithm of 1 is equal to zero, we can reexpress the above
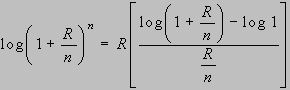
The key to the proof is to notice that the bracketed expression is a difference quotient for the logarithm function. So
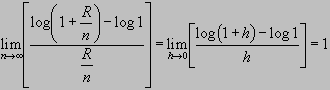
From elementary calculus, we know that
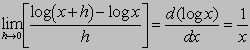
and in our case, x=1. This implies that

and consequently,
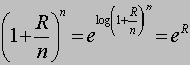
Example
Suppose a bank offers a nominal interest rate of 4% (R=.04) on your savings deposit. The following table illustrates the different effective or true interest rates depending on how many times the deposit is compounded each year.



So the investor will always prefer more compounding intervals to less. The continuous time rate of interest is always higher than the periodic interest rate.
The future value formula is derived by example. Suppose you have a deposit of $1000 which is paid 4% annually. What is the deposit's value at the end of six years?
Year Beginning Interest Ending Balance
1 1000.00 40.00 1040.00 2 1040.00 41.60 1081.60 3 1081.60 43.26 1124.86 4 1124.86 44.99 1169.85 5 1169.85 46.79 1216.64 6 1216.64 48.67 1265.31
The formula for future value is straight-forward from this example:
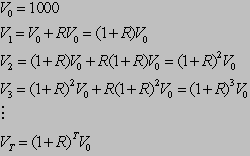
So the future value of today's deposit V_0 at the end of T periods is (1+R)^TV_0. If the deposit earns interest at the continuous time rate of interest, the formula is
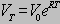
For the above example the future value of the $1000 invested at a continuous time rate of interest is $1271.25. Note again that the future value will be higher when continuous time compounding is used because the effective or true rate of interest is higher.
The term or is sometimes referred to as the accumulation factor or the money multiplier.
As the name implies, the money multiplier measures the factor by which your money multiplies in the future given an interest rate R and a maturity T.
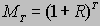
Often the return on an investment depends upon the length of time the money is tied up. Consider a schedule of bank interest rates. The 1-5 year rates were quoted from Wachovia.
Investment Money Period Rate (R) Multiplier (M_T)
1 year 8.150 1.0815000 2 year 8.200 1.1707240 3 year 8.350 1.2719989 4 year 8.400 1.3807566 5 year 8.500 1.5036567 . .
10 year 9.00 2.3673637 15 year 9.00 3.6424824 20 year 9.00 5.6055107 30 year 9.00 13.267678
Note that the money market multiplier increases exponentially with longer time to maturity. Furthermore, the rate of growth depends upon the interest rate.
Suppose we know the future value (V_T) of an investment. The present value of that investment (V_0) is easily calculated. From the formula for future value, we know:
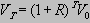
Divide both sides by the accumulation factor or the money multiplier and we get the present value:
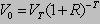
In continuous time, we have the following formula:
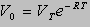
Example
A person needs $200,000 ten years from now to put her child through the WWW MBA. How much should she invest today, to pay off $200,000 in the future if the rate of interest she can lock in is 8%?
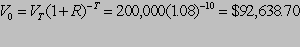
We can use the tools that we have developed to calculate present value and future value to examine zero coupon bonds. A zero coupon bond is a bond that pays $1 at time T and no coupons prior to this period. They are traded in the U.S. with names like zeros, money multipliers, CATs, TIGRs, and STRIP. CATs are Salomon Bros' Certificates of Accrual on Treasury Securities. TIGRs are Merrill Lynch's Treasury Investment Growth and Receipts and STRIPS are Separate Trading of Registered Interest and Principal of Securities.
These securities sell at a substantial discount from their par value of $1. The discount represents the interest earned on the investment through its life. Now let's derive the formula to get the yield from the price of a zero. From present value, we know how to bring back a future cash flow to the present with a given interest rate. From future value, we know how to bring forward today's cash flow with a given interest rate. For calculating the yield, we have the present value (the price of the bond) and the future value (the par price of the bond at maturity) and we solve for the interest rate. Consider the interest rate formula:
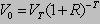
Divide both sides by the present value and by the inverse of the money multiplier:
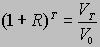
Now move the time to maturity to the right hand side:
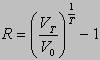
This is the formula for calculating the yield to maturity of a zero coupon bond. In continuous time, the formula is
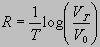
Example
Suppose a CAT that matures six years from now is trading for $55 and suppose the par value is $100. The yield can be calculated as:

The continuous time yield is:
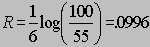
Note that the continuous time yield is always lower.
As an example of a bond price schedule, consider the quotations for CATs (Certificates of Accrual on Treasury Bills) that are drawn from Wall Street Journal.
Maturity Date Price
3 year 74.63 5 years 52.00 12 years 41.00 16 years 32.00 17 years 28.00 19 years 18.75
Note that the prices of the bonds declines with time.
See Zero coupon price dynamics
We can easily link the money multiplier formula to zero coupon bonds. Consider the following illustration. Suppose a 12 year zero sells for $.25 (and pays $1.00 in 12 years). If the investor has $1.00 to invest today, he can buy 4 zeros. So $1.00 invested today will yield $4.00 in twelve years. The money multiplier is 4.
The zeros and the money multipliers are related as follows:
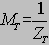
where Z_T is the price of the zero today and M_T is the money multiplier for T periods. Writing out the formula for the multiplier we have:

The immediate consequences are:
Higher interest rates imply
higher money multipliers
and
lower zero coupon bond prices.
See Zero coupon price dynamics
Suppose that you have a riskless investment that will produce the following cash flows over the next 5 years:
CF_1=10
CF_2=20
CF_3=30
CF_4=40
CF_5=50
What is the present value of this investment?
To answer this question, we can think of the cash flows as a portfolio of zero coupon bonds that are maturing in years 1 through 5. We can construct a replicating portfolio by purchasing zeros with $1 par price.
Cash #of Zeros' Time Year Flow Zeros to Maturity
1 10 10 1 2 20 20 2 3 30 30 3 4 40 40 4 5 50 50 5
Suppose that there existed prices for the zero coupon bonds for every maturity we are concerned with. We can then exactly replicate the cash flows with the zero coupon bonds:
Example: Valuing Cash Flows from Zero Coupon PricesZero Coupon Payoffs 10 20 30 40 50 Entire Year 1-yr 2-yr 3-yr 4-yr 5-yr Portfolio
1 10 0 0 0 0 10 2 0 20 0 0 0 20 3 0 0 30 0 0 30 4 0 0 0 40 0 40 5 0 0 0 0 50 50
Price 0.885 0.783 0.693 0.613 0.543 (per unit) Replication 8.85 15.66 20.79 24.52 27.14 96.96 Cost
So for an outlay of $96.96 today, you go into the market and buy zero coupon bonds that will give you the same payoffs at the same dates as your investment. This is way of looking at the present value of a stream of cash flows. Previously, we considered one cash flow at period T and we derived a method to bring it back to today. Clearly, we can think of that method as buying zeros today that replicate the cash flows.
We can value cash flows by creating an alternative portfolio of traded assets which exactly replicates the cash flows of the project. By the principle of no arbitrage, the replicating portfolio must have the same value as the project. Equal cash flows in all the different states of the world must have the same value. If one has a higher value, its price would be bid up as investors try to take advantage of the arbitrage opportunity. The lower value investment would have selling pressure as investors dump it and go to the higher value investment. The prices equalize as a result.
It is no always possible to find zero coupon bonds that can be used to replicate the project. Actually, the prices used in the previous section were derived from the assumption that the interest rate was 13%. Consider the same cash flows:
Cash Zero Coupon Price Present Value of Year Flow assuming 13% year's cash flow
1 10 (1.13)^-1=.885 8.85 2 20 (1.13)^-2=.783 15.66 3 30 (1.13)^-3=.693 20.79 4 40 (1.13)^-4=.613 24.52 5 50 (1.13)^-5=.543 27.14
Net Present Value = 96.96
Note that the net present value that we derived here is exactly the same as the one we derived before. The reason being that the zero coupon prices we used were exactly 13% zero coupon prices.
We can use the same technology to value an investment project or an acquisition being considered by a firm. For an investment project, it will likely have both costs and revenues. Net present value is the value of the investment to you today -- net of costs, i.e.,
NPV=PV(revenues)-PV(costs)
Consider the following scenario. You sit on the board of a firm that is considering an acquisition. The plan is to buy the firm, rationalize their operation and inject your firm's expertise in their production operations, and then sell after only two years. The cost today is $100 million. A profit of $10 million will be recorded at the end of one year. The firm will then be sold for $160 million. The discount rate that the firm uses is 10%. Ignore (for now) tax considerations. The net present value of the project is:
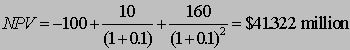
One point of fundamental importance is that any positive net present value project can be self- financed, i.e., the project pays itself for all the costs. Moreover, once carried out, the positive net present value is the gain measured in dollars today. To see it, we examine a strategy for the previous example. Suppose you are able to borrow for two years $100 million from the bank at 10%. You purchase the firm in question. You pay the first year interest with the revenue from the project. At the end of the second year, you owe the bank $110 million and you have sold the project for $160. The net gain in year 2 is $50 million. In today's dollars, the value is $41.322.
Remember, in the transactions we have executed, none of our capital has been touched. However, our wealth today has increased by $41.322 million. It should come clear that:
The Net-Present-Value Rule
(a) If a project has a positive net present value, it should be taken; if it has negative NPV, it should be
rejected;
(b) Among a group of mutually exclusive positive net present value projects, only the one
with the highest NPV should be taken.
We will have more to say about project evaluation later in the course.
Of considerable interest is how the present value of the cash flows from a project or the present value of the cash flows from a bond investment vary with changes in the interest rate. Since we have expressed the present value in terms of the interest rate and the cash flows, the direction of change can be determined by the first derivative:
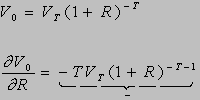
Equivalently, in terms of the zero coupon bond:
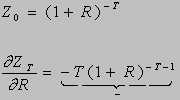
Note that the sign of these derivatives is negative. This means that the price of the zero coupon bond or the present value of the cash flow will decrease with an increase in the interest rate. Notice that the time to maturity, T, affects the rate of decrease. A longer term zero coupon bond will decrease by more than a short term zero coupon bond.
To illustrate these points, go to Zero coupon price dynamics or Bond price dynamics. Note that the prices always drop with higher interest rates. The price decline is rapid during the first few years and levels off as maturity increases to 30 years.
It is useful to go through an example at this point. Consider a coupon paying bond that has five years to maturity. The coupon is 12% and interest is paid annually. Let us determine the present value of this bond under two interest rate scenarios: 12% and 13%.
Cash (12% Rate) (13% Rate) Year Flow Zeros PV Zeros PV
1 12 .893 10.71 .885 10.62 2 12 .797 9.57 .783 9.40 3 12 .712 8.54 .693 8.32 4 12 .636 7.63 .613 7.36 5 112 .567 63.55 .543 60.82
Bond Price 100.00 96.52
As the interest rate goes up, the price of the bond decreases. At a 12% interest rate, the bond is valued exactly at par -- but if the rate increases to 13%, the coupon stream and the final payment of the principal becomes less valuable to the investor and hence the price decreases.
A valuable measure of bond performance is holding period return. This measures the rate of return on holding an asset over a particular amount of time. This measure takes both the change in the price of the bond as well as the coupon into account. For example, suppose a 6 year (original maturity) 12% coupon bond were bought at par ($100) and held for one year. Assume the coupon is paid once a year. Suppose at the end of the holding period, the interest rate had risen from 12% to 13%. The rate of return can be calculated as:
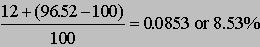
So while the investor made 12% on the coupon payment, the drop in the price of the bond caused the holding period return to decrease to 8.53%.
An annuity is a stream of cash flows that are equally spaced in time and of equal amount. An example is a $70,000 mortgage at 14%/12=1.166% that is paid off with a 180 month annuity of $932.22. We will show how to calculate the present value of an annuity and how to determine the size of an annuity that is necessary to pay off a certain present value (like the $70,000 mortgage).
First, we need to introduce some definitions:

Suppose we consider an annuity of $1 per period. We know how to discount each period back by calculating the price of a zero coupon bond.
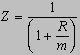
So the present value of an n period annuity can be calculated by summing the discounted cash flows:

It is tedious to go through and sum all of these term. The expression can be simplified by the rule of summation of geometric series. The trick to this rule is to multiply the sum by Z and then subtract the two quantities.

If the annuity is not $1 per period, then we can follow the steps again and the determine the value of the annuity to be:
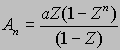
where a is the size of the annuity payment. We can also put this formula explicitly in terms of the interest rates. Let i equal the effective periodic rate of interest R/m.

Note that if the number of payments becomes infinite, then the present value of the annuity simplifies:
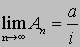
An example of an annuity with an infinite number of constant payments is the British consol bond. It pays a coupon at the end of each year and never matures.
Now we will return to the example of the $70,000 mortgage. Suppose you borrow $70,000 and repay over 15 years. The interest rate is 14% annually and payments are made monthly. The effective periodic rate of interest is 14/12=1.167% per month. Let us solve for the monthly payment "a" that is needed to pay off the mortgage. From our formula for the present value of the annuity, we know:
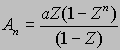
The strategy will be to substitute in for all the variables that we know (A_n, n, Z) and solve for the one variable that we do not know (a). First, we know that the present value must be $70,000. Second, calculate the zero coupon price:

Now let's consider another example. This will highlite the idea of an amortization schedule. Suppose that $1000 is borrowed. The loan will be repaid in 5 equal annual payments (each includes interest and principal). The interest rate is 10% per annum. First, compute the one period zero coupon bond price:
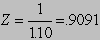
Now plug into the formula

Now we can check the mechanics by constructing an amortization schedule:
Amortization Schedule Beginning 10% Principal Ending Year Balance Interest Payment Part Balance
1 1000.00 100.00 263.80 163.80 836.20 2 836.20 83.62 263.80 180.18 656.02 3 656.02 65.60 263.80 198.20 457.82 4 457.82 45.78 263.80 218.02 239.80 5 239.80 23.98 263.80 239.82 000.00
This example illustrates the accounting implications of using a annuity. Note that there is a 2 cent rounding error.
Bonds usually pay a standard coupon amount, C, at regular intervals and this represents the interest on the bond. At the maturity of the bond, the final interest payment is made plus the principal amount (or par amount) is repaid.
U.S. Treasury bonds and notes pay interest semi-annually, e.g., in May and November. A bond with a quoted annual coupon of 8.5% really makes 8.5/ 2 or $4.25 per $100 of bond value twice a year.
Bond traders quote prices as percent of par, with fractions in 32nds. For example, a price of 102-8 on a bond means 102.25% of par. If the par amount is $10 million, then the price is $10,225,000.
Consider the time line of the payoffs of a 4 year, 8% T-note.
Today Maturity Time 0 6 12 18 24 30 36 42 48
Coupon 0 4 4 4 4 4 4 4 4 Principal 0 0 0 0 0 0 0 0 100
The thing that is obvious when we look at the time line is that we can value the bond by taking the present value of the coupon annuity and the present value of the final principal payment.
We will now introduce the general formula for pricing the bonds. First, we need some more notation:

Viewing the price of the bond as a sum of the present value of the coupon annuity and the present value of the final payment, we can derive the price of the bond:

Now we will calculate the price of a few bonds. Suppose that the current stated rate is 12.5% compounded semi-annually. There are two bonds in the market both mature in 12 years. Bond A has a 8.75% coupon rate (compounded semi-annually) and Bond B has a 12.625% coupon rate (compounded semi-annually). Before we start the calculations, it is clear that Bond B should be more valuable than Bond A. The coupon rate on Bond B is above the fair rate in the market and we expect it to be selling at a premium (above par). On the other hand, Bond A has a lower coupon rate and should be selling at a discount (below par). First, calculate the value of the one period zero.
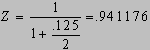
The value of the 24 period zero also needs to be calculated to bring the principal back to present value.

Now calculate the value of a one dollar annuity (a=$1).
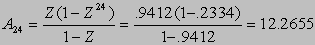
The coupons are easily calculated:
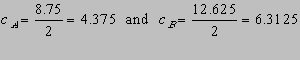
Now we can plug into our bond valuation formula:

The yield to maturity or the internal rate of return solves the following:
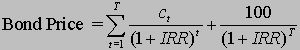
In the previous examples, we were given the fair interest rate, and were interested in calculating the price. Now we are given the bond price, and are asked to calculate the yield to maturity.
We can also view the internal rate of return as the rate that makes the bond present value less the bond price
exactly equal to zero.
There is no easy way to calculate the internal rate of return. Usually a computer will solve the equation
numerically. [Excel has an IRR function which solves the equation numerically.] One should also
remember that the internal rate of return assumes a flat term structure and that future rates are known. There
are advantages and disadvantages to using the IRR. The first is that we are solving for the interest rate rather
than plugging one in. Second, it is a widely used measure, i.e., reported in the press.
Now we will illustrate some of the problems in using the IRR. Suppose we have 2 bonds: Bond A and Bond
B. Suppose they both cost $1000.
Note that both of the bonds have the same scale costing $1000. Furthermore, they have the same investment
horizon of 3 years. It appears as if Bond A is better -- having a higher yield. But this is not necessarily the
case.
Suppose the
term structure was not flat. In particular, suppose we are facing the following term structure.
The one period forward interest rates are:
First year = i_1 = 10%
Second year = f_2 = 20%
Third year = f_3 =15%
Now calculate the present value:
From these calculations, the future value of Bond B is greater than Bond A and the present value of Bond B
is greater than Bond A.
It is clear from this example that Bond B is a superior investment to Bond A. If we vary the shape of the
term structure, then the IRR rule will not always work.
We have shown that the price of the bond is sensitive to the interest rate. Another factor that has to be taken
into account when ranking bonds is the time shape of the cash flows. If Bond B's cash flows are
concentrated in the far future, then its price will be very sensitive to changes in interest rates. Conversely, if
Bond A's cash flows are concentrated in the near future, it will not be as sensitive to changes in the interest
rate.
Consider the following example.
Now calculate the present values of these cash flows for various discount rates.
So the time path of cash flows is very important. Graphically, the present values of Bond A and Bond B
appear below.
We will say that Bond B has a greater duration than Bond A.
See Bond price dynamics.
A forward interest rate is a marginal return for investing your money for an extra period, i.e.,
investing for t periods rather than t-1 periods. The annualized forward rate between period 1
and 2can be written:
Solving for the forward rate is quite easy.
We can also calculate multiperiod (annualized) forward rates. The forward rate between years 1 and 3 is:
Finally, if the interest rates are compounded semiannually, then the annualized forward rate between
years 3 and 4 is
Notice that I have used i for the effective periodic rate and a lower case f to denote the
forward rate over a period less than one year.
There is an important alternative interpretation to the forward rate. Suppose we look in the paper and find a
1 year zero (face value $100) trading at $92.59 (yield of 8% annual rate no compounding) and a 2 year zero
trading at $79.72 (yield of 12% annual rate no compounding). Consider the following strategy. We sell
or short $100 million face value of the one year bond. We use the proceeds ($92.59) to purchase as
much of the 2 year as possible. At the end of the first year, we invest $100 million of our own money to pay
off what we owe (cover the short). At the end of the second year, we realize the revenue from cashing in the
two year bonds.
Let's go through the mechanics. First, what does it mean to short? Technically, I sell something to you that I
don't have. You want to buy a 1 year zero. You can buy from the government for $92.59. You get a bond
certificate. Next year you redeem it for $100. You post a gain. Alternatively, you pay me $92.59. I give you
a piece of paper saying that you get the official certificate next year. Next year I purchase a certificate from
the government for $100 (because there is no time to maturity) and hand it over to you. Actually, I would
probably just hand you the $100. This is called covering the short position.
So what about our example. We short $100 million face value of the one year. In doing this, we get paid
$92.59 million. We use this to buy the 2 year. We are able to purchase {$92,592,590/$79.71938}=
1,161,480 of these bonds. At the end of one year, we cover the short position by investing $100 million. At
the end of two years, we redeem the bonds for $116,148,000. The one year return from years one to two is
(116.148-100)/100=16.148%. This is exactly the forward rate from years one to years two. Hence, the
forward rate is also the return to an investment strategy which involves shorting and buying bonds of
different maturities.
The term structure of interest rates or the yield curve is the relation between yields observed today on
bonds of different maturity. The yield curve is upward sloping if longer term bonds have higher yields than
shorter term bonds or Treasury bills. The curve is flat if all the yields are approximately the same. The
structure is inverted if yields on short term bills are higher than long term bonds.
There have been many theories proposed to explain the term structure of interest rates. As the graph below
shows, it is quite variable. The three main theories that you probably studied in your macro course are:
expectations, liquidity preference and preferred habitat. The expectations theory just says that a positively
sloped yield curve means that investors expect rates to go up. Liquidity preference suggests that a rate
premium is attached to longer term bonds because they are more volatile. The preferred habitat says that
different rates across different maturities are due to differential demand by investors for particular
maturities.
There are problems with all of these theories. Consider an alternative story. The yield curve tells us about
future economic prospects. If the yield curve is inverted, it is because people are giving up their short term
investments and locking in longer term investments to weather a potential recession. This is just hedging. In
the good state of the economy, you give up some wealth to hedge against a potential recession. You prefer
smooth consumption expenditures to volatile ones. This theory is presented in my thesis paper Harvey
(1988, Journal of Financial Economics) [P1] and applied in many other papers such as Harvey
(1991, Journal of Fixed Income [P6].
I find that an inverted yield curve has preceeded every recession in the last 25 years. Consider the most
recent business cycle (which is an out-of-sample test of my theory). The yield curve inverted in the summer
of 1989. The inversion was mild (less than one percent) and lasted three quarters. The official peak of the
business cycle was July 1990 and the trough March 1991. The yield curve predicted the timing, duration and
magnitude of the recession.
See The Term Structure of Interest
Rates.
We have calculated what happens to bond price when there is an interest rate change. Consider the same
an example that we previously pursued:
Cash (12% Interest) (13% Interest) (14% Interest)
Year Flow Zeros PV Zeros PV Zeros PV
As the interest rate goes up, the price of the bond decreases. At a 12% interest rate, the bond is valued
exactly at par -- but if the rate increases to 13%, the coupon stream and the final payment of the principal
becomes less valuable to the investor and hence the price decreases. The decline is even more severe when
the rate jumps to 14%. The holding period return also decreases. If this was a six year bond bought at par
and held for one year, then the return on holding the bond is 8.52% if the rates go to 13% and 5.13% if the
rates go to 14%. You can imagine that bigger swings in the rates could cause negative holding period
returns. In this case, the capital loss exceeds the gain from collecting the coupon. The longer the maturity of
the bond the more severe the price changes when the yield changes.
A measure of the volatility of the bond price movement is valuable. We will consider two measures:
duration and elasticity. Both of these measures will give us local approximations, i.e., they will
be accurate for only small movements in the rates.
First, let's review the formula for bond price.
where B represents the price of the bond. Note that the cash flows can represent coupon payments
and principal. The logical way to measure the sensitivity of the bond price to changes in the interest rate is
to take the first derivative of B with respect to r. We can write the bond price formula:
If we adjust this measure by dividing by the bond price and multiplying by one plus the market yield, we
get a measure of duration first introduced by Macaulay in 1938.
Duration was invented as alternate measure of the timing of the cash flows from bonds. The pitfall in using
the maturity of a bond as a measure of timing is that it only takes into consideration the final payment of the
principal -- not the coupon payments. Macaulay suggested using the duration as an alternative measure that
could account for all of the expected cash flows. Duration is a weighted average term to maturity where the
cash flows are in terms of their present value. We can rewrite the above equation in a simpler format:
where PVCF_1 measures the present value of the cash flow in period one and PVTCF measures the
present value of all the cash flows or the bond price.
Now lets consider examples of duration calculations. We will calculate the duration of Bond A and Bond B.
The market interest rate is assumed to be 8%. Both bonds have a maturity of 10 years. Bond A has a coupon
of 4% and Bond B has a coupon of 8%. Before we calculate the duration measure, we know that Bond B
will have a shorter duration. The cash flows from 1--9 years are larger yet the principal is identical. Now
lets work it out.
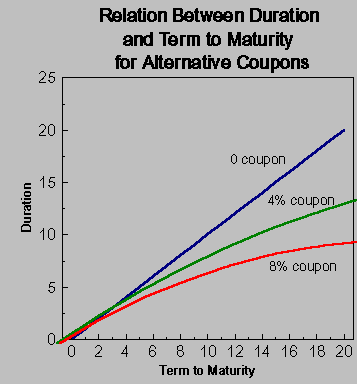
As expected, the bond with the higher coupon rate has a shorter duration. This example illustrates two
important properties of duration. First, the duration of a bond is less than its time to maturity (except for
zero coupons). Second, the duration of the bond decreases the greater the coupon rate. This can be
graphically illustrated:
Notice that the duration and maturity are identical for the zero coupon and the duration decreases with
higher coupon rates. This is because more weight (Present Value Weight) is being given to the coupon
payments.
The final property is that, as market yields increase, the duration of the bond decreases. This should be
intuitively obvious because when we are discounting cash flows a higher discount rate means a lower
weight on cash flows in the far future. Hence, the weighing of the cash flows will be more heavily placed on
the early cash flows -- decreasing the duration.
The link between duration and volatility is clear because of the first derivative that we used in obtaining the
duration formula. There are two alternative measures that are worth investigating. The first is called
modified duration. This is derived by dividing the duration measure by one plus the current market
yield.
There is also a measure known as elasticity. This is calculated as:
The elasticity measure will be very close in practice to the modified duration measure. The closeness is
dependent upon the size of the movement in interest rates. Hence, we see the link between duration,
modified duration, and elasticity. Now lets consider another example. We will look at bonds of different
maturity. Bond A has a 10 year maturity with a 12% coupon and Bond B has a 5 year maturity with a 12%
coupon. Suppose that the market discount rate is 13%.
Note that the price elasticity measure is very close to the modified duration measure. Note also that the
closeness of this measure depends upon the size of the interest rate move. If we go back and calculate the
elasticity for our previous example of a bond with a 4% coupon and the relevant discount rate is 8% -- the
denominator is now 4% and the approximation will be less accurate.
See Bond price dynamics.
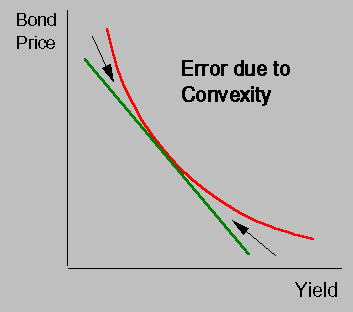
We would like to use the modified duration to approximate bond price movements for a given change in
interest rates. The approximation will only be accurate for small changes in the interest rate. For large
changes, such as 5%, the approximation deteriorates. This is because the bond price is convex in the
yields. We have seen this convexity when we looked at the plot of the bond price against various yields to
maturity. Below is a figure that illustrates the error.
Also, if stated interest rates are compounded semi-annually, then the same procedure is used to calculate
duration in half years. The duration in half years is converted to years (by dividing by 2)
and then transformed into modified duration by divided by the
(effective periodic semi-annual rate).
One might wonder why we divide by the semi-annual rate rather than (1) the
annual percentage rate or (2) the true annualized rate. The answer, unfortunately,
has to do with convention. Most top investment houses calculate the modified duration
by dividing by the semi-annual rate - (even if there is quarterly or monthly
compounding). In most of my examples, I have annual compounding so I don't have
to worry about this convention.
Below is a numerical example using annual rates. Suppose we have two bonds with identical coupon rates
of 10%. Bond A has a maturity of 5 years and Bond B has a maturity of 10 years. Let's estimate the error of
the duration approximation for price change for a 5% increase in the discount rate.
Bond A Bond B
The reason for this error is the bond price convexity. In large interest rate moves, this convexity is
increasingly important. Convexity is measure of the way the slope of the bond price -- yield curve is
changing. Duration gives us a linear approximation. But if the curve is convex this linear approximation
may not be very high quality.
To get convexity, expand the bond price formula:
The general formula for a Taylor Series expansion is:
If this is not familiar, you can review your undergraduate calculus text. It should have a section on Taylor
series. Take f(x) to be the pricing function for the bond. The price is a function of the yield
(x). Consider the change in the yield to be the hvariable. I cut off the approximation after the
second term. The first order part, f'(x), is associated with the duration of the bond and the second
order part f''(x) with the convexity of the bond. There will also be a residual part because
the Taylor series is only an approximation. He provides tables in which he measures how well each of these
parts accounts for price changes for bonds of various maturities. Note that the duration measure that he uses
is modified duration.
Suppose, for investment purposes, you own $10 million in 8% 30-year Treasuries. The modified duration is
12. The market value is exactly at par, $10 million. However, you believe rates will move up over the next
year. If you do nothing and rates move up from 8% to 9%, you stand to lose $1.2 million in market value on
your investment. You don't want to sell the bonds - but you would like to protect yourself from this potential
loss over the next year. This where we would hedge.
Suppose you plan to buy a house in the next two years. You know that mortgage rates are at 15 year lows
and you want to lock in a rate. If you do nothing and rates move up, you will be faced with much more
expensive financing. You would like to protect yourself against this potentially higher cost. By hedging, we
can lock the mortgage rate in today.
The goal of hedging is to provide a positive cash flow in the bad state. In the first example, our hedge will
deliver a payoff that offsets the $1.2 million loss after one year. In the second example, the hedge will
deliver a payoff if mortgage rates go up. Even though you finance your mortgage at the higher rate, you are
compensated by a positive payoff from the hedge.
We usually use futures or options to execute our hedges. In our first example, there is a 20-year bond
contract trading on the CBOT. The bond has a
duration of approximately 8.00. We would agree to sell $15 million or 150 contracts. Roughly speaking (and
we will go into more details later), you can think of this as generating $15 million cash flow -- like any short
position. Suppose rates increase next year to 9%. The bond price drops by 8% (the duration) to $13.8
million. To cover the short, you need only $13.8 million. The gain is $1.2 which exactly offsets our loss on
the bonds we hold. In a futures contract, the $15 million inflow and the $13.8 million outflow are never
physically executed -- you just get the difference.
Note we sold $15 million in the futures. How did I get that number? We know that the bond we hold is 50%
more volatile than the bond in the futures. Since I hold $10 million in the 30 year bond, I must sell $15
million in the futures (50% more). The exact formula begins with a calculation of how much you will lose
(unhedged) for a 1% move in rates.
because the market value of the CBOT contract is $100,000. A=150. Of course, if the rates move
the other way, the gain on what we hold is wiped out by the loss in the futures. We have effectively locked
in the 8% rate.
This is our first example of hedging. In actual practice, investors care about matching convexity (and
sometimes skewness and kurtosis).
My BA453 Tactical Global Asset
Allocation provides an indepth analysis of hedging techniques.
Additional information is available in Value and Risk Management Through Derivatives.
Much of the materials for this lecture are from Douglas Breeden, "Interest Rate Mathematics", Robert
Whaley, "Derivation and Use of Interest Formulas" and Campbell R. Harvey and Guofu Zhou, "The Time
Value of Money".
BA 350
Index Page
Bond A Bond B
Price 1000 1000
Cash Flows:
Year 1 145 430
Year 2 145 430
Year 3 1145 430
Yield or IRR 14.5% 13.9%
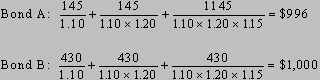
Bond IRR PV FV
A 14.5% 996 1512
B 13.9 1000 1518 15. Bond Rankings and Interest Rates
Bond A Bond B
Year Cash Flows Cash Flows
1 263.80 0
2 263.80 0
3 263.80 0
4 263.80 0
5 263.80 1611.51
Discount
Rate PV(A) PV(B) Better
0% 1320 1611 B
5% 1142 1262 B
10% 1000 1000 -
15% 884 801 A
20% 789 647 A

16. Forward Interest Rates
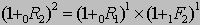
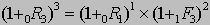

17. The Term Structure of Interest
Rates
18. Bond Price Movements and Duration
Example
1 12 .893 10.71 .885 10.62 .877 10.53
2 12 .797 9.57 .783 9.40 .769 9.23
3 12 .712 8.54 .693 8.32 .675 8.10
4 12 .636 7.63 .613 7.36 .592 7.10
5 112 .567 63.55 .543 60.82 .519 58.17
Bond Price 100.00 96.52 93.13
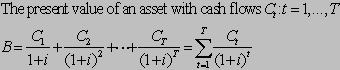


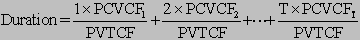
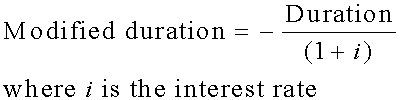
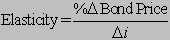
Rate 10 Year Bond 5 Year Bond
Year 13% Cash PV PV/Tot Cash PV PV/Tot
1 .885 12 10.62 .11 12 10.62 .11
2 .783 12 9.40 .10 12 9.40 .10
3 .693 12 8.32 .09 12 8.32 .09
4 .613 12 7.36 .08 12 7.36 .07
5 .543 12 6.52 .07 112 60.82 .63
6 .481 12 5.77 .06
7 .425 12 5.10 .05
8 .376 12 4.51 .05
9 .333 12 4.00 .04
10 .294 112 32.93 .35
94.53 1.00 96.52 1.00
Duration= 6.22 years 4.02 years
Modified Duration = 6.22/1.13 = 5.50 4.02/ 1.13 = 3.56
Elasticity=[(100-94.53)/100]/1.00=5.47% [(100-96.52)/100]/1.00=3.48%
Example
Bond Maturity 5 years 10 years
Coupon Rate 10% 10%
Modified Duration 3.79 6.14
(at par 10%)
Predicted Bond
Price Move -18.95% -30.72%
Actual Bond
Price Move -16.76% -25.09%
Error of
Duration Approx 2.19% 5.63%

19. Duration and Hedging

Acknowledgement
Campbell Harvey's Home Page